Quantum repetition code for phase-flips#
The code presented in the previous notebook could only correct for $X$ errors. Now, we present a code that can only correct for $Z$ errors. In the next notebook, we will combine these codes together to correct for both $X$ and $Z$ errors and more.
Setup#
Alice wants to send a one-qubit state $\ket{\psi}$ to Bob, but via a channel that applies the $Z$ operator to qubits with probability $p$. If the error occurs then the state is distorted as, \begin{equation} \ket{\tilde\psi} = Z\ket{\psi} = Z(\alpha\ket{0} + \beta\ket{1}) = \alpha\ket{0} - \beta\ket{1}. \end{equation} Bob cannot tell if an error has occurred, so with probability $p$ he will receive the wrong message.
Alice and Bob therefore use an error-correcting procedure.
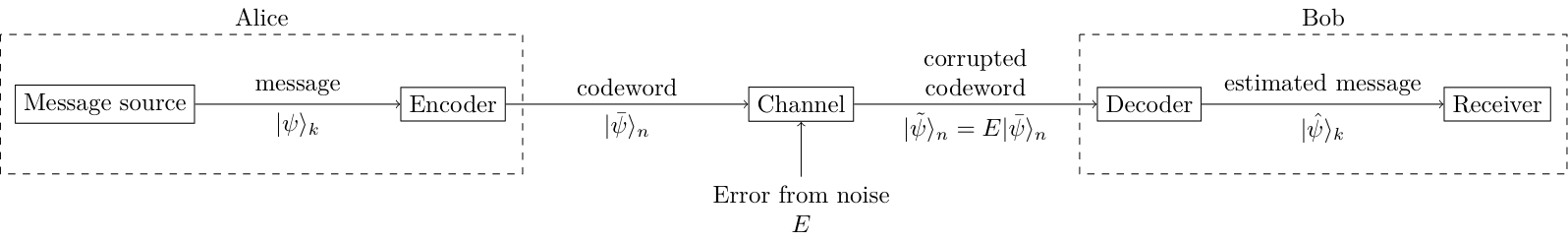
Here we assume that on each qubit, $Z_i$ occurs with probability $p$.
Encoding#
To protect against such type of errors, we will employ a repetition code, but with a different basis. Now, \begin{align} \ket{0} \to \ket{\bar{0}} = \ket{+++}, \\ \ket{1} \to \ket{\bar{1}} = \ket{---}. \end{align} For this code basis, a qubit in state $\ket{\psi}$ is encoded as \begin{equation} \ket{\psi}\ket{00} = (\alpha\ket{0} + \beta\ket{1})\ket{00} \to \ket{\bar\psi} = \alpha\ket{+++} + \beta\ket{---}. \end{equation} This is done via the following circuit.
import stac
enc_circ = stac.Circuit.simple(3)
enc_circ.append('CX', 0, 1)
enc_circ.append('CX', 0, 2)
for i in range(3):
enc_circ.append('H', i)
enc_circ.draw()Task 1 (On paper)#
Determine logical gate operations $\bar{X}$ and $\bar{Z}$ for the three-qubit repetition code for phase-flips. These are operations that act on the logical basis $\set{\ket{\bar{0}}, \ket{\bar{1}}}$, in the normal way, i.e. \begin{align} \bar{X}\ket{\bar{0}} &= \ket{\bar{1}}, \quad \bar{X}\ket{\bar{1}} = \ket{\bar{0}}, \\ \bar{Z}\ket{\bar{0}} &= \ket{\bar{0}}, \quad \bar{Z}\ket{\bar{1}} = -\ket{\bar{1}}. \end{align} They can be constructed by some combination of operations on the three physical qubits. You will discover that there are possibly multiple ways of doing so.
Errors#
The possible errors on the encoded state are given as follows.
| Error | Probability |
|---|---|
| $I$ | $(1-p)^3$ |
| $Z_0$ | $p(1-p)^2$ |
| $Z_1$ | $p(1-p)^2$ |
| $Z_2$ | $p(1-p)^2$ |
| $Z_0Z_1$ | $p^2(1-p)$ |
| $Z_0Z_2$ | $p^2(1-p)$ |
| $Z_1Z_2$ | $p^2(1-p)$ |
| $Z_0Z_1Z_2$ | $p^3$ |
Task 2 (On paper)#
Determine the impact of each error on the input basis states.
Let input state be $\ket{+++}$
| Error | Output state |
|---|---|
| $I$ | $\ket{+++}$ |
| $Z_0$ | $\ket{-++}$ |
| $Z_1$ | $?$ |
| $Z_2$ | $?$ |
| $Z_0Z_1$ | $?$ |
| $Z_0Z_2$ | $?$ |
| $Z_1Z_2$ | $?$ |
| $Z_0Z_1Z_2$ | $?$ |
Let input state be $\ket{---}$
| Error | Output state |
|---|---|
| $I$ | $\ket{---}$ |
| $Z_0$ | $\ket{+--}$ |
| $Z_1$ | $?$ |
| $Z_2$ | $?$ |
| $Z_0Z_1$ | $?$ |
| $Z_0Z_2$ | $?$ |
| $Z_1Z_2$ | $?$ |
| $Z_0Z_1Z_2$ | $?$ |
Do different errors result in the same output state?
As before, we will assume that $p$ is small, so we will only attempt to correct the errors $\set{I,Z_0,Z_1,Z_2}$. The effect of the $Z$ errors is to flip the state between plus and minus. So, for instance, \begin{equation} Z_0\ket{\bar{\psi}} = \alpha Z_0\ket{+++} + \beta Z_0\ket{---} = \alpha\ket{-++} + \beta\ket{+--}. \end{equation}
Decoding#
The error detection strategy is the same as before. Bob employs a circuit that compares the value of two pairs of qubits, but in the plus/minus basis. This is accomplished using the following circuit.
synd_circ_expanded = stac.Circuit.simple(5)
synd_circ_expanded.append('H', 0)
synd_circ_expanded.append('CX', 0, 3)
synd_circ_expanded.append('H', 0)
synd_circ_expanded.append('TICK')
synd_circ_expanded.append('H', 1)
synd_circ_expanded.append('CX', 1, 3)
synd_circ_expanded.append('H', 1)
synd_circ_expanded.append('TICK')
synd_circ_expanded.append('H', 1)
synd_circ_expanded.append('CX', 1, 4)
synd_circ_expanded.append('H', 1)
synd_circ_expanded.append('TICK')
synd_circ_expanded.append('H', 2)
synd_circ_expanded.append('CX', 2, 4)
synd_circ_expanded.append('H', 2)
synd_circ_expanded.append('TICK')
synd_circ_expanded.append('M', 3)
synd_circ_expanded.append('M', 4)
synd_circ_expanded.draw()Here in each block, first we use the Hadamard gate to switch to the computational basis, then transfer the value of the qubit to the ancilla qubit using the $CX$ gate. Finally, we convert back to the plus/minus basis using the Hadamard gate. We can simplify this circuit as follows, using the fact that $H^2 = I$.
synd_circ = stac.Circuit.simple(5)
for i in range(3):
synd_circ.append('H', i)
synd_circ.append('TICK')
synd_circ.append('CX', 0, 3)
synd_circ.append('CX', 1, 3)
synd_circ.append('CX', 1, 4)
synd_circ.append('CX', 2, 4)
synd_circ.append('TICK')
for i in range(3):
synd_circ.append('H', i)
synd_circ.append('TICK')
synd_circ.append('M', 3)
synd_circ.append('M', 4)
synd_circ.draw()To determine the syndromes, we can do the following
circ = stac.Circuit.simple(5)
# encode the 0 state
circ += enc_circ
# # add an error
circ.append('TICK')
circ.append('Z', 0)
circ.append('TICK')
# # do a syndrome measurement
circ += synd_circ
# draw to make sure you we understand what is happening
circ.draw()
# sample the output 10 times
circ.sample(10)[1 0] [1 0] [1 0] [1 0] [1 0] [1 0] [1 0] [1 0] [1 0] [1 0]
Task 3#
Fill the following table
| Syndrome | Inferred error |
|---|---|
| $00$ | $I$ |
| $01$ | |
| $10$ | |
| $11$ |
In this code, if Bob infers that errors $Z_i$ occurs, than he applies $Z_i$ to the corrupted codeword to fix it.
Bit-flip errors#
We won't belabor the point that this code cannot fix bit-flip errors.